Pertanyaan

Perhatikan daerah yang diarsir berikut! Nilai minimum dari fungsi tujuan dengan rumus f ( x , y ) = 2.000 x + 3.000 y untuk daerah yang diarsir adalah ...

Perhatikan daerah yang diarsir berikut!

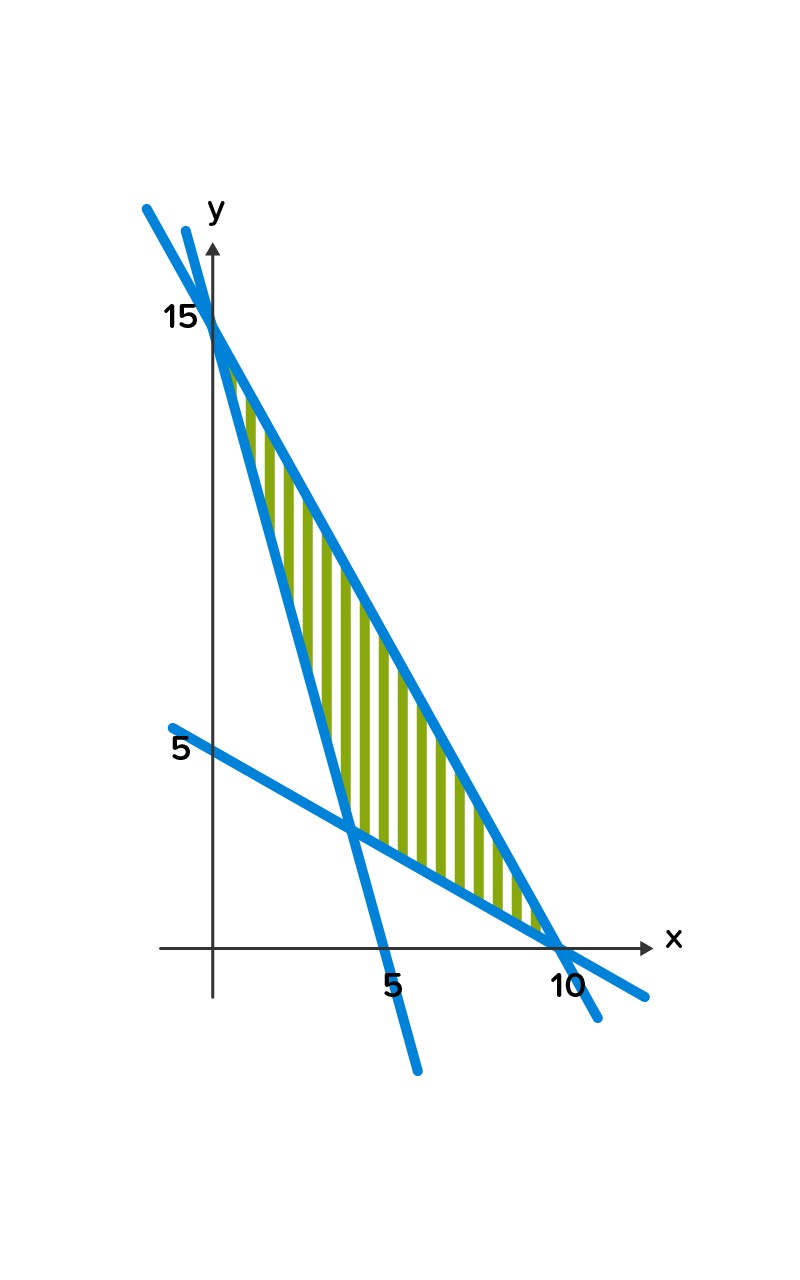
Nilai minimum dari fungsi tujuan dengan rumus $f (x, y) = 2.000 x + 3.000 y$ untuk daerah yang diarsir adalah ...

Ikuti Tryout SNBT & Menangkan E-Wallet 100rb

Habis dalam

Klaim

E. Dwi

Master Teacher

Mahasiswa/Alumni Universitas Sriwijaya

Jawaban terverifikasi

Jawaban

nilai minimum dari fungsi tujuan dengan rumus untuk daerah yang diarsir adalah .

nilai minimum dari fungsi tujuan dengan rumus f left parenthesis x comma y right parenthesis equals 2.000 x plus 3.000 y

untuk daerah yang diarsir adalah 17.000

Pembahasan

Diketahui: Pertidaksamaa dari dua garis yang masing-masing melalui titik . Titik potong dua pertidaksamaan tersebut: Substitusikan nilai untuk menentukan nilai . Maka, titik potong dari dua pertidaksamaan adalah . Dengan menggunakan metode titik pojok, maka nilai minimum dari fungsi tujuan dapat ditentukan sebagai berikut: Jadi, nilai minimum dari fungsi tujuan dengan rumus untuk daerah yang diarsir adalah .

Diketahui:

open parentheses 5 comma 0 close parentheses space dan thin space left parenthesis 0 comma 15 right parenthesis left parenthesis 10 comma 0 right parenthesis space dan space left parenthesis 0 comma 5 right parenthesis open parentheses 10 comma 0 close parentheses space dan space left parenthesis open parentheses 0 comma 15 close parentheses

Pertidaksamaa dari dua garis yang masing-masing melalui titik open parentheses 5 comma 0 close parentheses space dan space open parentheses 0 comma 15 close parentheses space serta space open parentheses 10 comma 0 close parentheses space dan space open parentheses 0 comma 5 close parentheses .

open parentheses 5 comma 0 close parentheses space dan space open parentheses 0 comma 15 close parentheses rightwards double arrow 15 x plus 5 y greater or equal than 75 open parentheses 10 comma 0 close parentheses space dan space open parentheses 0 comma 5 close parentheses rightwards double arrow 5 x plus 10 y greater or equal than 50

Titik potong dua pertidaksamaan tersebut:

Substitusikan nilai untuk menentukan nilai .

table attributes columnalign right center left columnspacing 0px end attributes row cell 5 x plus 10 y end cell equals 50 row cell 5 open parentheses 4 close parentheses plus 10 y end cell equals 50 row cell 20 plus 10 y end cell equals 50 row cell 10 y end cell equals 30 row y equals cell 30 over 10 end cell row y equals 3 end table

Maka, titik potong dari dua pertidaksamaan 15 x plus 5 y greater or equal than 75 space dan space 5 x plus 10 y greater or equal than 50 adalah open parentheses 4 comma 3 close parentheses .

Dengan menggunakan metode titik pojok, maka nilai minimum dari fungsi tujuan f left parenthesis x comma y right parenthesis equals 2.000 x plus 3.000 y dapat ditentukan sebagai berikut:

Jadi, nilai minimum dari fungsi tujuan dengan rumus f left parenthesis x comma y right parenthesis equals 2.000 x plus 3.000 y untuk daerah yang diarsir adalah 17.000 .

Perdalam pemahamanmu bersama Master Teacher
di sesi Live Teaching, GRATIS!

5.0 (1 rating)

Pertanyaan serupa

Seorang pemilik toko sandal memiliki modal Rp4.000.000,00. Ia membeli setiap pasang sandal A Rp10.000,00 dan sandal B Rp8.000,00. Setiap pasang sandal A dan sandal B masing-masing memberi keuntungan R...

4.3

Jawaban terverifikasi

Nilai maksimum dari f ( x , y ) = 6 x + 4 y yang memenuhi sistem pertidaksamaan 2 x + y ≤ 4 , x + y ≤ 3 , x ≥ 0 , dan y ≥ 0 adalah ...

4.9

Jawaban terverifikasi

Nilai minimum dari bentuk ( 3 x + y ) pada daerah penyelesaian sistem pertidaksamaan 2 x + y ≥ 4 ; x + y ≥ 3 ; x ≥ 0 ; y ≥ 0 adalah...

4.7

Jawaban terverifikasi

Nilai minimum fungsi objektif f(x,y) = 5x + 6y yang memenuhi sistem pertidaksamaan 2 x + y ≥ 8 , 2 x + 3 y ≥ 12 , x ≥ 0 , y ≥ 0 ; x , y ∈ R adalah ....

5.0

Jawaban terverifikasi

Perhatikan gambar berikut. Nilai minimum fungsi objektif f ( x , y ) = y − 2 x dicapai di titik ....

5.0

Jawaban terverifikasi