Pertanyaan

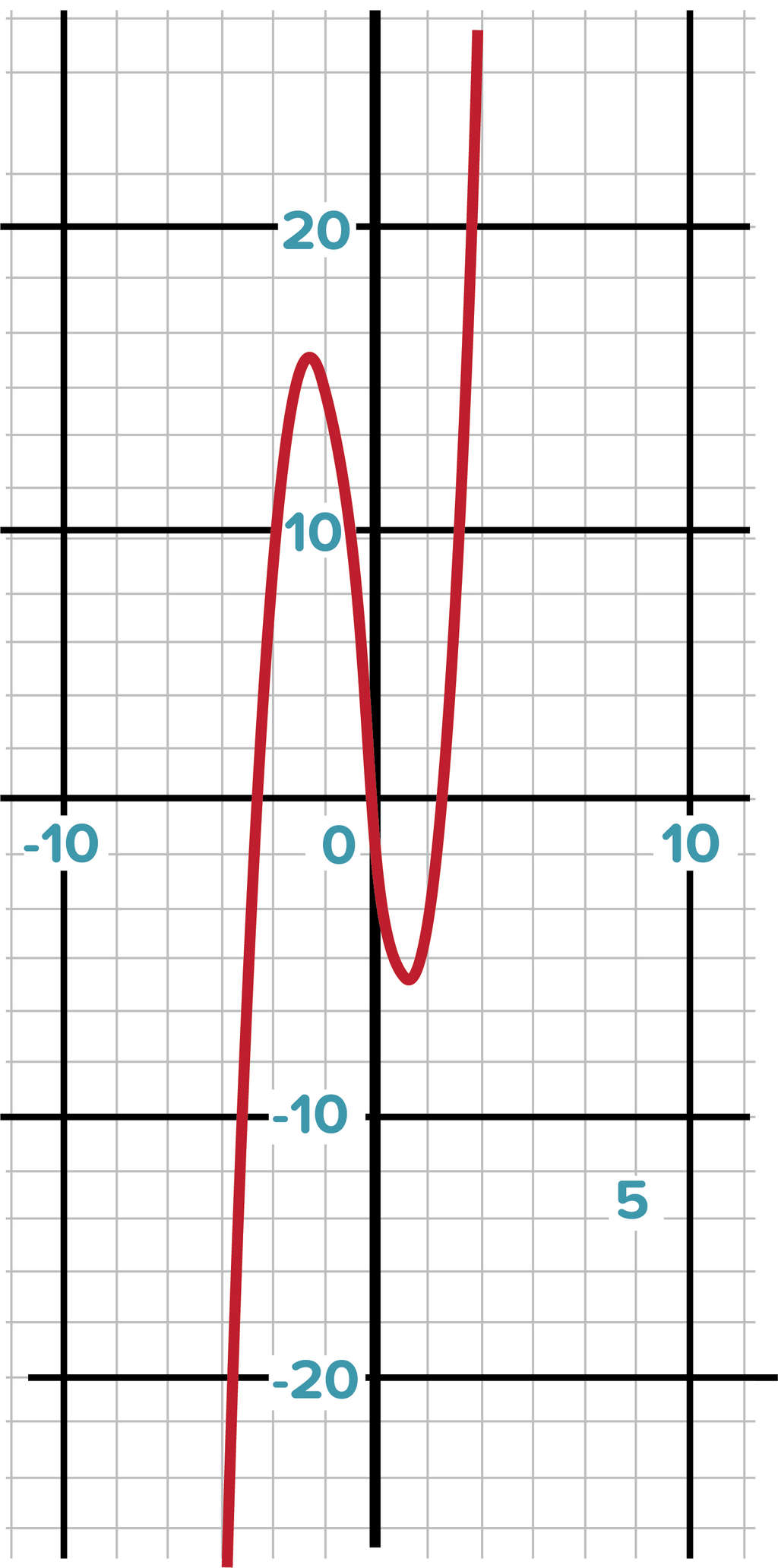
Gambarlah sketsa grafik dari fungsi berikut! 3. x ( x − 2 ) ( x + 4 )

Gambarlah sketsa grafik dari fungsi berikut!

3. $x (x - 2) (x + 4)$

I. Kumaralalita

Master Teacher

Mahasiswa/Alumni Universitas Gadjah Mada

Jawaban terverifikasi

Pembahasan

Diberikan fungsi atau dapat dituliskan . Titik potong sumbu didapatkan dengan mensubstitusikan . Titik potong sumbu didapatkan dengan mensubstitusikan . . Dengan demikian, kurva tersebut memotong sumbu-sumbu koordinat di titik . Titik stasioner didapatkan dengan turunan pertama fungsi, yaitu . Dengan syarat stasioner maka . Akan dihitung akar-akar persamaan kuadrat Substitusikan akar-akar persamaan ke fungsi awal. Jenis stasioner diperoleh menggunakan turunan kedua fungsi, yaitu . Subtitusikan titik stasioner ke persamaan turunan kedua, didapatkan : . Karena maka titik merupakantitik balik minimum. Sedangkan maka titik merupakan titik balik maksimum. Jadi, sketsa grafik tersebut adalah

Diberikan fungsi f left parenthesis x right parenthesis equals x left parenthesis x minus 2 right parenthesis left parenthesis x plus 4 right parenthesis atau dapat dituliskan .

Titik potong sumbu negative x didapatkan dengan mensubstitusikan y equals 0 .

f left parenthesis x right parenthesis equals 0 x left parenthesis x minus 2 right parenthesis left parenthesis x plus 4 right parenthesis equals 0 x equals 0 space logical or space x equals 2 space logical or x equals negative 4

Titik potong sumbu negative y didapatkan dengan mensubstitusikan x equals 0 .

f left parenthesis 0 right parenthesis equals 0 cubed plus 2 times 0 squared plus 8 times 0 equals 0 .

Dengan demikian, kurva tersebut memotong sumbu-sumbu koordinat di titik left parenthesis negative 4 comma space 0 right parenthesis comma space left parenthesis 0 comma space 0 right parenthesis comma space dan space left parenthesis 2 comma space 0 right parenthesis .

Titik stasioner didapatkan dengan turunan pertama fungsi, yaitu f apostrophe left parenthesis x right parenthesis equals 3 x squared plus 4 x minus 8 . Dengan syarat stasioner maka . Akan dihitung akar-akar persamaan kuadrat

$table attributes columnalign right center left columnspacing 0px end attributes row cell x subscript 1 comma 2 end subscript end cell equals cell fraction numerator negative b plus-or-minus square root of b squared minus 4 a c end root over denominator 2 a end fraction end cell row blank equals cell fraction numerator negative 4 plus-or-minus square root of 16 minus 4 times 3 times left parenthesis negative 8 right parenthesis end root over denominator 2 times 3 end fraction end cell row blank equals cell fraction numerator negative 4 plus-or-minus square root of 16 plus 96 end root over denominator 6 end fraction end cell row blank equals cell fraction numerator negative 4 plus-or-minus square root of 112 over denominator 6 end fraction end cell row blank equals cell fraction numerator negative 4 plus-or-minus 4 square root of 7 over denominator 6 end fraction end cell row cell x subscript 1 end cell equals cell fraction numerator negative 4 plus 4 square root of 7 over denominator 6 end fraction equals 1 comma 097 end cell row cell x subscript 2 end cell equals cell fraction numerator negative 4 minus 4 square root of 7 over denominator 6 end fraction equals negative 2 comma 431 end cell end table$

Substitusikan akar-akar persamaan ke fungsi awal.

Jenis stasioner diperoleh menggunakan turunan kedua fungsi, yaitu f apostrophe apostrophe left parenthesis x right parenthesis equals 6 x plus 4 . Subtitusikan titik stasioner ke persamaan turunan kedua, didapatkan :

Karena f apostrophe apostrophe left parenthesis 1 comma 097 right parenthesis greater than 0 maka titik left parenthesis 1 comma 097 comma negative 5 comma 049 right parenthesis merupakan titik balik minimum. Sedangkan maka titik left parenthesis negative 2 comma 431 comma space 16 comma 901 right parenthesis merupakan titik balik maksimum.

Jadi, sketsa grafik tersebut adalah