Iklan
Pertanyaan
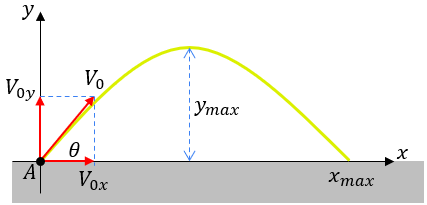
Dari titik A di tanah, sebuah bola dilemparkan dengan kecepatan awal 20 m/s dan sudut elevasi 37° (sin 37° = 0,6). Jika g = 10 m/s², hitunglah: Komponen kecepatan awal dalam arah horizontal dan vertikal, Kecepatan bola setelah 0,4 sekon, Posisi bola setelah 0,4 sekon, Tinggi maksimum yang dapat dicapai bola, dan Jarak lemparan terjauh yang dicapai bola.
Dari titik A di tanah, sebuah bola dilemparkan dengan kecepatan awal 20 m/s dan sudut elevasi 37° (sin 37° = 0,6). Jika g = 10 m/s², hitunglah:
- Komponen kecepatan awal dalam arah horizontal dan vertikal,
- Kecepatan bola setelah 0,4 sekon,
- Posisi bola setelah 0,4 sekon,
- Tinggi maksimum yang dapat dicapai bola, dan
- Jarak lemparan terjauh yang dicapai bola.
Iklan
YM
Y. Maghfirah
Master Teacher
Jawaban terverifikasi
17
4.7 (18 rating)
YI
Yusup Iskandar Manalu
Makasih ❤️
RL
Reno Letwart
Jawaban tidak sesuai
AP
Alexander Pandey
Mudah dimengerti
PP
Pabuaran Pandu
Pembahasan lengkap banget
Iklan
Pertanyaan serupa
Tanya ke AiRIS
Yuk, cobain chat dan belajar bareng AiRIS, teman pintarmu!
RUANGGURU HQ
Jl. Dr. Saharjo No.161, Manggarai Selatan, Tebet, Kota Jakarta Selatan, Daerah Khusus Ibukota Jakarta 12860
Produk Ruangguru
Bantuan & Panduan
Hubungi Kami
©2025 Ruangguru. All Rights Reserved PT. Ruang Raya Indonesia