Pertanyaan

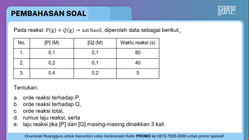
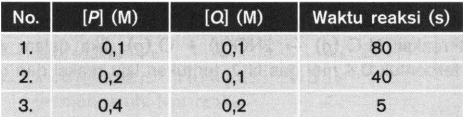
Pada reaksi: P ( g ) + Q ( g ) → zat hasil , diperoleh data sebagaiberikut. Tentukan: a. orde reaksi terhadap P, b. orde reaksi terhadap Q, c. orde reaksi total, d. rumus laju reaksi, serta e. laju reaksi jika [ P ] dan [ Q ] masing-masing dinaikkan 3kali.

Pada reaksi: $P (g) + Q (g) \to zat hasil$ , diperoleh data sebagai berikut.

Tentukan:

a. orde reaksi terhadap P,

b. orde reaksi terhadap Q,

c. orde reaksi total,

d. rumus laju reaksi, serta

e. laju reaksi jika $[P]$ dan $[Q]$ masing-masing dinaikkan 3 kali.

Ikuti Tryout SNBT & Menangkan E-Wallet 100rb

Habis dalam

Klaim

I. Solichah

Master Teacher

Jawaban terverifikasi

Jawaban

orde P, orde Q,orde total, rumuslaju reaksi, dan perubahan laju jika konsentrasi dinaikkan 3kali berturut-turut adalah 1, 2, 3, , dan 9kali lebih cepat dibandingkan laju awal.

orde P, orde Q, orde total, rumus laju reaksi, dan perubahan laju jika konsentrasi dinaikkan 3 kali berturut-turut adalah 1, 2, 3, r double bond k open square brackets P close square brackets open square brackets Q close square brackets squared

r double bond k open square brackets P close square brackets open square brackets Q close square brackets squared

, dan 9 kali lebih cepat dibandingkan laju awal.

Pembahasan

Hukum laju reaksi menyatakan sebuah persamaan yang memperlihatkan keterkaitan atau hubungan antara laju reaksi tertentu dengan konsentrasi pereaksinya. Berdasarkan persamaan reaksi , maka persamaan laju reaksi dapat ditulis menjadi: dimana m dan n adalah orde masing-masing reaktan. Penentuan orde reaksi masing-masing reaktan dapat dicari berdasarkan data eksperimen dengan cara membandingkan data laju reaksi dan konsentrasi reaktan yang diketahui. Konsep yang perlu kita gunakan juga adalah hubungan waktu (t) terhadap laju reaksi (r). Langkah 1: Tentukan orde X dan orde Y. Langkah 2: Tentukan orde total. Langkah 3: Tentukan rumus laju reaksi. Substitusi nilai m = 1 dan n = 2ke dalam rumus laju reaksi. Langkah 4: Tentukanlaju reaksi jika dan masing-masing dinaikkan 3kali. Dengan demikian, diperoleh hasil bahwa: a. orde reaksi terhadap P= 1. b. orde reaksi terhadap Q= 2. c. orde total = 3. d. rumus laju reaksi, . e. laju reaksi jika dan masing-masing dinaikkan 3kali menjadi 9kali lebih cepat dibandingkan laju awal. Jadi, orde P, orde Q,orde total, rumuslaju reaksi, dan perubahan laju jika konsentrasi dinaikkan 3kali berturut-turut adalah 1, 2, 3, , dan 9kali lebih cepat dibandingkan laju awal.

Hukum laju reaksi menyatakan sebuah persamaan yang memperlihatkan keterkaitan atau hubungan antara laju reaksi tertentu dengan konsentrasi pereaksinya.

Berdasarkan persamaan reaksi P open parentheses italic g close parentheses and Q open parentheses italic g close parentheses yields zat space hasil , maka persamaan laju reaksi dapat ditulis menjadi:

r double bond k open square brackets P close square brackets to the power of m open square brackets Q close square brackets to the power of n

dimana m dan n adalah orde masing-masing reaktan.

Penentuan orde reaksi masing-masing reaktan dapat dicari berdasarkan data eksperimen dengan cara membandingkan data laju reaksi dan konsentrasi reaktan yang diketahui. Konsep yang perlu kita gunakan juga adalah hubungan waktu (t) terhadap laju reaksi (r).

r almost equal to 1 over t

Langkah 1: Tentukan orde X dan orde Y.

$table attributes columnalign right center left columnspacing 0px end attributes row blank blank cell bold Orde bold space P space... space perc point space left parenthesis 1 right parenthesis space dan space left parenthesis 2 right parenthesis end cell row cell k subscript 1 over k subscript 2 open square brackets P subscript 1 close square brackets to the power of m over open square brackets P subscript 2 close square brackets to the power of m open square brackets Q subscript 1 close square brackets to the power of n over open square brackets Q subscript 2 close square brackets to the power of n end cell equals cell r subscript 1 over r subscript 2 end cell row cell k subscript 1 over k subscript 2 open square brackets P subscript 1 close square brackets to the power of m over open square brackets P subscript 2 close square brackets to the power of m open square brackets Q subscript 1 close square brackets to the power of n over open square brackets Q subscript 2 close square brackets to the power of n end cell equals cell fraction numerator 1 over t subscript 1 over denominator 1 over t subscript 2 end fraction end cell row cell open parentheses fraction numerator 0 comma 1 over denominator 0 comma 2 end fraction close parentheses to the power of m open parentheses fraction numerator 0 comma 1 over denominator 0 comma 1 end fraction close parentheses end cell equals cell fraction numerator 1 over 80 over denominator 1 over 40 end fraction end cell row cell open parentheses 1 half close parentheses to the power of m end cell equals cell 40 over 80 end cell row cell open parentheses 1 half close parentheses to the power of m end cell equals cell open parentheses 1 half close parentheses to the power of 1 end cell row m equals 1 row blank blank blank row blank blank cell bold Orde bold space italic Q space... space perc point space left parenthesis 2 right parenthesis space dan space left parenthesis 3 right parenthesis space left parenthesis Substitusi space m equals 1 right parenthesis end cell row cell fraction numerator k subscript 2 over denominator k 3 end fraction open square brackets X subscript 2 close square brackets to the power of m over open square brackets X subscript 3 close square brackets to the power of m open square brackets Y subscript 2 close square brackets to the power of n over open square brackets Y subscript 3 close square brackets to the power of n end cell equals cell r subscript 2 over r subscript 3 end cell row cell fraction numerator k subscript 2 over denominator k 3 end fraction open square brackets P subscript 2 close square brackets to the power of m over open square brackets P subscript 3 close square brackets to the power of m open square brackets Q subscript 2 close square brackets to the power of n over open square brackets Q subscript 3 close square brackets to the power of n end cell equals cell fraction numerator 1 over t subscript 2 over denominator 1 over t subscript 3 end fraction end cell row cell open parentheses fraction numerator 0 comma 2 over denominator 0 comma 4 end fraction close parentheses to the power of 1 open parentheses fraction numerator 0 comma 1 over denominator 0 comma 2 end fraction close parentheses to the power of n end cell equals cell fraction numerator 1 over 40 over denominator 1 fifth end fraction end cell row cell open parentheses 1 half close parentheses open parentheses 1 half close parentheses to the power of n end cell equals cell 5 over 40 end cell row cell open parentheses 1 half close parentheses to the power of n end cell equals cell 5 over 40 division sign 1 half end cell row cell open parentheses 1 half close parentheses to the power of n end cell equals cell 5 over 40 cross times 2 over 1 end cell row cell open parentheses 1 half close parentheses to the power of n end cell equals cell 10 over 40 end cell row cell open parentheses 1 half close parentheses to the power of n end cell equals cell 1 fourth end cell row cell open parentheses 1 half close parentheses to the power of n end cell equals cell open parentheses 1 half close parentheses squared end cell row n equals 2 end table$

Langkah 2: Tentukan orde total.

table attributes columnalign right center left columnspacing 0px end attributes row cell orde space total end cell equals cell orde space P and orde space Q end cell row cell orde space total end cell equals cell 1 plus 2 end cell row cell orde space total end cell equals 3 end table

Langkah 3: Tentukan rumus laju reaksi. Substitusi nilai m = 1 dan n = 2 ke dalam rumus laju reaksi.

Langkah 4: Tentukan laju reaksi jika open square brackets P close square brackets dan open square brackets Q close square brackets masing-masing dinaikkan 3 kali.

$table attributes columnalign right center left columnspacing 0px end attributes row cell open square brackets P close square brackets subscript akhir end cell equals cell 3 cross times open square brackets P close square brackets subscript awal end cell row cell open square brackets P close square brackets subscript akhir end cell equals cell 3 open square brackets P close square brackets end cell row cell open square brackets Q close square brackets subscript akhir end cell equals cell 3 cross times open square brackets Q close square brackets subscript awal end cell row cell open square brackets Q close square brackets subscript akhir end cell equals cell 3 open square brackets Q close square brackets end cell row cell laju space akhir space open parentheses r subscript 1 close parentheses end cell equals cell... ? end cell row blank blank blank row cell r subscript 1 over r end cell equals cell fraction numerator k open square brackets P close square brackets subscript akhir open square brackets Q close square brackets subscript akhir over denominator k open square brackets P close square brackets open square brackets Q close square brackets end fraction end cell row cell r subscript 1 over r end cell equals cell fraction numerator k cross times 3 open square brackets X close square brackets cross times 3 open square brackets Y close square brackets over denominator k cross times open square brackets X close square brackets cross times open square brackets Y close square brackets end fraction end cell row cell r subscript 1 over r end cell equals cell fraction numerator 3 cross times 3 over denominator 1 end fraction end cell row cell r subscript 1 over r end cell equals cell 9 over 1 end cell row cell r subscript 1 end cell equals cell 9 cross times r end cell end table$

Dengan demikian, diperoleh hasil bahwa:

a. orde reaksi terhadap P = 1.

b. orde reaksi terhadap Q = 2.

c. orde total = 3.

d. rumus laju reaksi, r double bond k open square brackets P close square brackets open square brackets Q close square brackets squared .

e. laju reaksi jika open square brackets P close square brackets dan open square brackets Q close square brackets masing-masing dinaikkan 3 kali menjadi 9 kali lebih cepat dibandingkan laju awal.

Jadi, orde P, orde Q, orde total, rumus laju reaksi, dan perubahan laju jika konsentrasi dinaikkan 3 kali berturut-turut adalah 1, 2, 3, r double bond k open square brackets P close square brackets open square brackets Q close square brackets squared , dan 9 kali lebih cepat dibandingkan laju awal.

Perdalam pemahamanmu bersama Master Teacher
di sesi Live Teaching, GRATIS!

4.2 (7 rating)

Ratu Sabrina Turnip

Makasih ❤️

Patrisia Sirait

Mudah dimengerti Makasih ❤️

Eka Fitri

Makasih ❤️

Royhan Alamsyah

Jawaban tidak sesuai

Pertanyaan serupa

Pada percobaan reaksi gas nitrogen dan hidrogen dengan persamaan reaksi N 2 + 3 H 2 → 2 NH 3 didapatkan hasil percobaan sebagai berikut. Persamaan laju reaksi dari data di atas adalah …...

5.0

Jawaban terverifikasi

Disajikan data yang hasil percobaan reaksi: A + B → C sebagai berikut. Jika [A] dinaikkan 4 kali, [B] tetap, laju reaksi tetap. Jika [A] dan [B] masing-masing dinaikkan 2 kali, laju reaksi me...

3.6

Jawaban terverifikasi

Untuk reaksi P + Q → R + S , diperoleh data percobaan sebagai berikut: Tentukan: Orde terhadap zat P Orde terhadap zat Q Orde total Persamaan laju reaksi Tetapan laju reaksi...

5.0

Jawaban terverifikasi

Pada reaksi: 2 X ( a q ) + Y ( a q ) → Z ( a q ) diperoleh data percobaan sebagai berikut: Tentukan: orde reaksi total, rumus laju reaksi, nilai tetapan laju reaksi ( k ) dan satuann...

4.7

Jawaban terverifikasi

Suatu percobaan dilakukan pada reaksi: 2 CO ( g ) + O 2 ( g ) → 2 CO 2 ( g ) diperoleh data sebagai berikut. Berdasarkan data tersebut, pernyataan yang tepat adalah ...

4.6

Jawaban terverifikasi