Iklan
Pertanyaan
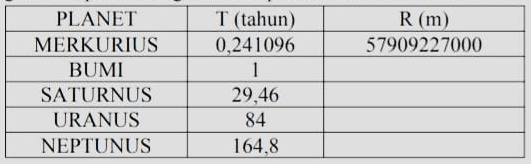
Hitunglah kecepatan mengorbit dari planet Neptunus dari data berikut ini!
Hitunglah kecepatan mengorbit dari planet Neptunus dari data berikut ini!
Iklan
YM
Y. Maghfirah
Master Teacher
Jawaban terverifikasi
1
0.0 (0 rating)
Iklan
RUANGGURU HQ
Jl. Dr. Saharjo No.161, Manggarai Selatan, Tebet, Kota Jakarta Selatan, Daerah Khusus Ibukota Jakarta 12860
Produk Ruangguru
Bantuan & Panduan
Hubungi Kami
©2025 Ruangguru. All Rights Reserved PT. Ruang Raya Indonesia















