Fatima N
13 Januari 2023 12:33
Iklan
Fatima N
13 Januari 2023 12:33
Pertanyaan
Perhatikan gambar di bawah ini! AC adalah garis singgung lingkaran M dengan titik singgung B. ∠MAB : ∠AMB = 2 : 3 dan ∠MCB : ∠BMC = 3 : 4. Tentukan besar masing-masing sudut yang belum diketahui!
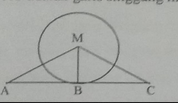
1
1
Iklan
R. Nurhayati
Mahasiswa/Alumni Universitas Negeri Semarang
25 Januari 2023 04:36
<p>Jawaban: ∠AMB = 54°, ∠MAB = 36°, ∠BMC≈ 51,43°</p><p>dan ∠MCB ≈ 38,57°.</p><p> </p><p> </p><p>Ingat konsep berikut:</p><p>Garis singgung lingkaran akan tegak lurus dengan jari-jari lingkaran yang melalui titik singgungnya dan membentuk sudut 90°.</p><p>Jumlah sudut dalam suatu segitiga adalah 180°.</p><p> </p><p>Diketahui AC adalah garis singgung lingkaran M dengan titik singgung B.</p><p>∠MAB : ∠AMB = 2 : 3 </p><p>Maka ∠MAB = 2/3 ∠AMB</p><p> </p><p>dan ∠MCB : ∠BMC = 3 : 4.</p><p>Maka ∠MCB = 3/4 ∠BMC</p><p> </p><p>Karena AC adalah garis singgung lingkaran, maka ∠ABM = ∠MBC = 90°.</p><p>Perhatikan segitiga ABM, maka:</p><p> ∠MAB + ∠AMB +∠ABM = 180°</p><p>2/3 ∠AMB + ∠AMB + 90° = 180°</p><p> 2/3 ∠AMB + ∠AMB = 90°</p><p> 2/3 ∠AMB + 3/3 ∠AMB = 90°</p><p> 5/3 ∠AMB = 90°</p><p> ∠AMB = 90° : (5/3)</p><p> ∠AMB = 90° x (3/5)</p><p> ∠AMB = 54°</p><p>Sehingga ∠MAB = 2/3 x 54° = 36°.</p><p> </p><p>Perhatikan segitiga BCM, maka:</p><p> ∠MCB + ∠BMC + ∠MBC = 180°</p><p> 3/4 ∠BMC + ∠BMC + 90° = 180°</p><p> 3/4 ∠BMC + 4/4 ∠BMC = 90°</p><p> 7/4 ∠BMC = 90°</p><p> ∠BMC = 90° : (7/4)</p><p> ∠BMC = 90° x (4/7)</p><p> ∠BMC = (360/7)°</p><p> ∠BMC≈ 51,43°</p><p>Sehingga</p><p>∠MCB = 3/4 x (360/7)°</p><p>∠MCB = (270/7)°</p><p>∠MCB ≈ 38,57°</p><p> </p><p>Jadi, besar sudut ∠AMB = 54°, ∠MAB = 36°, ∠BMC≈ 51,43°</p><p>dan ∠MCB ≈ 38,57°.</p>
Jawaban: ∠AMB = 54°, ∠MAB = 36°, ∠BMC≈ 51,43°
dan ∠MCB ≈ 38,57°.
Ingat konsep berikut:
Garis singgung lingkaran akan tegak lurus dengan jari-jari lingkaran yang melalui titik singgungnya dan membentuk sudut 90°.
Jumlah sudut dalam suatu segitiga adalah 180°.
Diketahui AC adalah garis singgung lingkaran M dengan titik singgung B.
∠MAB : ∠AMB = 2 : 3
Maka ∠MAB = 2/3 ∠AMB
dan ∠MCB : ∠BMC = 3 : 4.
Maka ∠MCB = 3/4 ∠BMC
Karena AC adalah garis singgung lingkaran, maka ∠ABM = ∠MBC = 90°.
Perhatikan segitiga ABM, maka:
∠MAB + ∠AMB +∠ABM = 180°
2/3 ∠AMB + ∠AMB + 90° = 180°
2/3 ∠AMB + ∠AMB = 90°
2/3 ∠AMB + 3/3 ∠AMB = 90°
5/3 ∠AMB = 90°
∠AMB = 90° : (5/3)
∠AMB = 90° x (3/5)
∠AMB = 54°
Sehingga ∠MAB = 2/3 x 54° = 36°.
Perhatikan segitiga BCM, maka:
∠MCB + ∠BMC + ∠MBC = 180°
3/4 ∠BMC + ∠BMC + 90° = 180°
3/4 ∠BMC + 4/4 ∠BMC = 90°
7/4 ∠BMC = 90°
∠BMC = 90° : (7/4)
∠BMC = 90° x (4/7)
∠BMC = (360/7)°
∠BMC≈ 51,43°
Sehingga
∠MCB = 3/4 x (360/7)°
∠MCB = (270/7)°
∠MCB ≈ 38,57°
Jadi, besar sudut ∠AMB = 54°, ∠MAB = 36°, ∠BMC≈ 51,43°
dan ∠MCB ≈ 38,57°.
· 0.0 (0)
Iklan
Mau pemahaman lebih dalam untuk soal ini?
Tanya ke Forum
Biar Robosquad lain yang jawab soal kamu

LATIHAN SOAL GRATIS!
Drill Soal
Latihan soal sesuai topik yang kamu mau untuk persiapan ujian


Perdalam pemahamanmu bersama Master Teacher
di sesi Live Teaching, GRATIS!



